A Dilated View of the Delayed Choice Quantum Eraser

Every so often, the DCQE (Delayed Choice Quantum Eraser) makes the rounds on various internet places. There are many good explanations of how it works and why it DOES NOT require weird explanations, like time travel.

However, these often seem to miss or gloss over a couple of important points about the Copenhagen Interpretation, which I'd like to talk about here. Following that, I'd like to present a thought experiment that shows that the different experimental configurations used to imply that there must be "something weird" going on are, in fact, all the same, and the results should not be a surprise and are in fact forced.

I'm going to assume you've seen some of this before and know at least roughly what things like photons and spin are.

A Quick Review of the DCQE

A DCQE is constructed by repeatedly trying to beat physics and failing. We start with the double slit experiment:

A laser is aimed at a screen through a closely spaced double slit, and the familiar double slit pattern from wave theory is cast on the screen. If either slit is closed, we get a single slit pattern on the screen.

Our first attempt to beat physics (the whole wave or particle thing) is done by turning the intensity of the laser down, either directly or by blocking some of the photons, and taking long exposures of the screen. Even if the intensity is so low that only single photons are emitted, we still get the same patterns, implying that an individual photon goes through both slits like a wave but hits the screen like a particle. (Note the images will be somewhat more fuzzy at low intensities, but that's not important right now).

Any attempt to determine which slit the photon traveled through results in either absorbing the photon early or the single slit pattern being observed, just as if one of the slits had been closed.

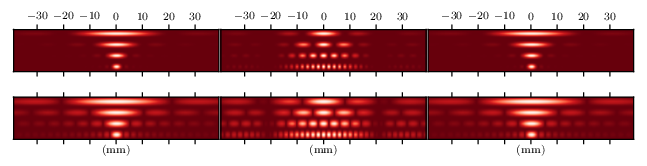
Next, we'll try to figure out which slit the photon particle went through. We use an optical crystal right after the slits to split the single photon into an entangled pair where energy and momentum are conserved (Note spin is not conserved, but that's not important right now). The photons in the pair travel on divergent trajectories that we'll call north and east. If we put screens on both trajectories, we'll get the double slit diffraction pattern on both screens. The hope is that we can keep a screen along the north trajectory to see the diffraction pattern while using the east trajectory to determine which slit the photon went through. Alas, this fails in that we only get the single slit diffraction patterns. This implies that the two photons are not independent but are correlated.

So, we can choose to either display the eastward photon on a screen or determine which slit it passed through, and our choice will affect the pattern observed on the northern screen. This implies that the photons are not merely correlated from their creation but continue to share a single wave function.

We can go a little further. We can make the choice to attempt to detect which slit was traversed random, using a 50/50 beam splitter, and instead of a screen, we can recombine the photon paths to 'erase' the traversal information in the same way a screen would. We'll call this complete device a 'decider,' and it outputs one of four results for each photon: R, L, X, or Y, depending on which detector the photon ends up triggering.

This is the full set up. We can measure the type of diffraction pattern on the northern trajectory and decide what type of measurement to make on the eastern. This decision can be either naturally random with beam splitters (as drawn) or electro-mechanical; the results are the same.

However, as we're dealing with individual photons, it takes many, many measurements to collect enough data. To see the correct patterns, each photon arriving at the northern screen has to be binned into an image based on the measurement result from the decider. The R and L images will be single slit patterns, while the X and Y images will be double slit patterns. The sum of all four images produces a washed-out single slit pattern.

Now for the Weirdness

As described, the diffraction pattern on the northern screen is dependent on choices or events in the decider. In the language of the Copenhagen interpretation: the decider's choice of measurement either does or does not collapse the wave function of the photon pair, resulting in the corresponding diffraction pattern seen at the screen.

However, if we move the northern screen close to the source and the decider far enough away from the source to know that a photon hits the screen long before its partner enters the decider, nothing changes. The results are exactly the same as if the decider is close to the source and the screen further away.

This leads to all sorts of odd explanations, often involving time travel or 'retrocausality'; after all, how else would a decision made after the photon hits the screen be able to effect what happens on the screen? And clearly, measuring one photon is having a non-local affect on the other photon! Often, an appeal is made to the fact that the patterns can only be seen after decoding to indicate that no real information is being passed between the entangled photons, so superluminal or retro-causal communication between the partners is fine!

Also, this is not where the weirdness is; it's a distraction!

To clean this up a bit, we first need to realize that entanglement is NOT unusual. It is, in fact, normal for particles to be entangled. It is removing all the entanglement to produce pure states that is hard and rare. Harder still is producing states that have just the correct amount of entanglement for some experiment.

Next ,we should swap the word 'measurement' for the word 'interaction', or rather use them interchangeably, and realize that a photon interacting with the screen is a measurement: we're measuring where the photon hits the screen. This makes our description of the experiment more symmetric. We have a photon that interacts with the screen on the northern trajectory and a photon that interacts with the decider on the eastern.

Lastly, when a wave function collapses, it doesn't collapse for every part of every wave function everywhere, but only for the part related to the interaction triggering the collapse. In doing so, it will also create new uncertainty in some other part. For example, if you measure the horizontal spin of an electron and then its vertical spin, the horizontal spin from the first measurement will be reset to a uniformly random state.

So it is better to say that a wave function collapses to a new function that is consistent with the measurement that was made. With this in mind, let's reexamine the experiment where one entangled photon interacts with the screen before the other interacts with the decider.

The mistake often made is to think that the wave function (and so its probabilities) of the photon going into the decider remain uniform and unchanged despite its partner having hit the screen. It doesn't. Interacting with the screen causes the wave function to collapse such that the probabilities for the R, L, X, and Y measurement is consistent with the location where the screen-photon interaction occurred. So if a photon hits the screen in a location that would normally be dark for a photon traversing just the L slit, then the function collapses such that the probability of the decider subsequently detecting a photon at the L detector is much lower.

So the situation is now completely symmetric with respect to how the arms of the experiment are configured. It doesn't matter how the screen and decider are arranged and which interacts first. The wave function always collapses to ensure consistent results.

And this is the weirdness: this consistent collapse happens non-locally, instantaneously, and everywhere in such a way that the result remains consistent and completely symmetric. It is not in the idea of entanglement per se. This non-locality was a problem way back in the first part of the experiment: the wave function has a 50%/50% chance of going through either slit, but the moment you measure or stop it at one slit, the probabilities at both slits instantaneously jump to either 100% or 0%, even though the two slits are by definition not in the same place. It's just that with entangled particles, you can move the interactions far enough apart that the issue becomes really freaking obvious.

But it gets weirder still, with some special relativity.

Let's set up a thought experiment with the northern screen and eastern decider at exactly \(10 m\) from the source, and we'll add four hypothetical observers: one stationary at the origin (call it 'O'), one traveling northward ('N'), one eastward ('E'), and one traveling along the center diagonal ('D'). N, E, and D are traveling at a comfortable \(99.5%\) of the speed of light, giving them a time dilation and length contraction factors of approximately \(10\).

Each observer will see the experiment configured differently. O will see both the screen and decider at \(10 m\), N will see the screen in close at \(1 m\) but the decider at \(10 m\), E will see the screen at \(10 m\) but the decider in close at \(1 m\), and lastly, D will see a symmetric configuration like O but at about \(7.1 m\).

While the observers will all disagree on things like distances, the frequency of the photons, simultaneity, and the ordering of non-local events like which photon hits first, they must agree on the overall results of the experiment. It doesn't matter whether a photon is observed hitting the screen first or the decider first; the screen location and decider's RLXY outputs must be the same for all the configurations and must decode the same way, because all the observers are observing the same experiment!

So... when does the collapse happen exactly? (This is a rhetorical question! This problem is known as 'The Measurement Problem').

Conclusion

Hopefully, this article has convinced you that the DCQE exhibits the normal level of quantum weirdness, but no more; There is nothing new going on, and there is no need to invoke weird retro time things. In the Copenhagen interpretation, every interaction is a measurement and vice versa, and it is important to properly update the wave function for every interaction so that the wave function remains consistent with each interaction. Lastly, the experiment must respect Special Relativity, and the disparate locations of the experiment's components mean different observers will see different orderings of events, and yet the results of the experiment must be the same for every observer.

None of this helps explain the measurement problem, but neither does it make it weirder. The problem exists without the DCQE. The DCQE, when viewed correctly, just puts a spotlight on one part. When viewed incorrectly, it just confuses things.